Summary
This multiple-day collaborative group lesson uses dice to explore probability of simple events similar to those in the book "Jumanji" by Chris Van Allsburg. Students will examine sample space, theoretical probability, and experimental probability and make predictions using outcomes of probability. (NOTE: Allow approximately two to three days to complete all activities and discussions. Estimated times are provided below in the lesson snapshot. These amounts may vary depending upon how much time is needed for class discussion, reflection, each activity, and length of each class period.)
Essential Question(s)
When might we use probability and why? Why do we need probability?
Snapshot
Engage
Students read the book Jumanji to engage and activate prior knowledge regarding likelihood of an simple event.
Explore
Students experiment with a partner to explore the probability of sums when rolling dice.
Explain
Students discuss and explain the connections and differences between sample space, theoretical probability, and experimental probability.
Extend
Students explore other games and activities recording sample space and theoretical probability for a game of students' choice. Engage in "game stations" that explore the experimental probability associated with games.
Evaluate
Students reflect through writing with a focus on learning sample space, theoretical probability, experimental probability and making predictions based on these concepts.
Materials
Lesson Slides (attached)
The book Jumanji by Chris Van Allsburg or access to the two Jumanji movie clips from YouTube
Dice (two per group)
Sums of Jumanji Recording Sheet (attached or in Google Drive; printed front and back; one per student)
Game Stations Probability Data Sheet (attached or in Google Drive; use for shared digital document)
Board games or other probability-based activities
Paper
Pencils
Engage
20 Minute(s)
Introduce the lesson using the attached Lesson Slides. Go to slide 3 to display the lesson's essential question: When might we use probability and why? Why do we need probability? Go to slide 4 to share the lesson's learning objective with students. Review this with your class to the extent you feel necessary.
Go to slide 5. Begin by asking if students have read the book or seen the movie Jumanji. If you have students who have read the book or seen the movie, ask one or two of them to provide a brief description of the story.
Go to slide 7. Read the book to the class, stopping on page 22 after Judy is told by Peter "If you roll a 12, you can get out of the jungle." (For video clip Jumanji 7/8, play the first 39 seconds, stopping the video right before Sarah rolls the dice.) Go to slide 8 for the book. Instruct students to use the Think-Pair-Share strategy to reflect on the question, "Do you think Judy is likely to roll a 12?" (Go to slide 9 for the video. Video question: "Do you think Sarah is likely to roll a seven to finish the game?") Students will reflect on the spectrum of likelihood (impossible, 0; unlikely, between 0 and 1/2; likely as not, 1/2; likely, between 1/2 and 1; or certain, 1.) Instruct students to turn to their Elbow Partners and take turns sharing and explaining their responses. Student pairs should then share with the whole class what they discussed (what they agreed or disagreed upon and why).
Go to slide 10. Finish reading the rest of the book. (Video: Finish watching Jumanji (7/8), and then go to slide 12 to show Jumanji (8/8). Ask the question, "What was the likelihood that Alan was going to roll a number that was three or greater?" Was the likelihood greater for Sarah or Alan to finish the game? Why?"). Go to slide 11 for the book. Return to the question about Judy and the game, but this time have students consider "Which sum or sums would Judy most likely have rolled with two standard dice?" (Video question: "Which sums were Sarah and Alan most likely to roll with two standard dice?") Have students Think-Pair-Share.
Go to slide 13. As a whole group discuss the question, "What sums are impossible to roll with two standard dice?" Allow students time to consider a few sums that would be impossible to create when rolling two standard dice. Students will hopefully come to a consensus that the sums can only be 2-12. Nothing less and nothing more would be possible unless they were to use dice with more sides or different numbers.
Go to slide 14. Briefly explain that the range of possible outcomes is called the Sample Space. In the story, the sample space are the possible sums.
Explore
15 Minute(s)
Go to slide 15. Students move into collaborative pairs, each receiving two dice and an attached Sums of Jumanji recording sheet. Before students begin rolling the dice, say to students, "Record a prediction at the top of your sheet: Which sum you think would appear most often and why?"
Go to slide 16. Ask each pair to roll the two dice 15 times. After each roll, students should record the value of the sum under the corresponding column. (See the image below for an example.)
After students complete 15 rolls, determine which sum appeared most frequently. Was it what they predicted? Take a moment to explore which sums theoretically should have appeared most frequently (6 and 8).
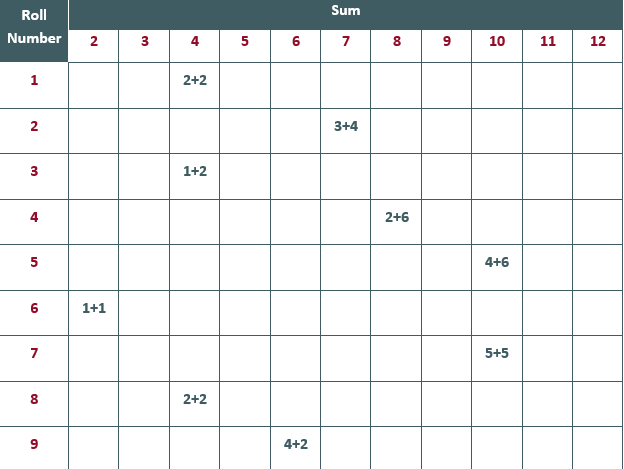
Go to slide 17. After student pairs have completed their rolls and recorded all data, instruct pairs to begin making predictions based on their data considering the scenarios below. These will be shared and explored further with the whole group during the Explain portion of the lesson.
"What might happen to the results if we continued to roll the dice another 10 times, 15 times, 100 times, etc.? What would you expect to happen to the sums?"
"If we rolled the dice ____ more times, how many times would you expect to get a sum that equals ____?"
"If we had rolled the dice only ____ times, how many times would you expect to have received a sum that equals ____?"
Student pairs should use proportional reasoning (or knowledge to make equivalent fractions or ratios) to consider and answer these questions. Each group will receive different answers based on their experimental data. Also, for the groups who cannot solve for a whole number, discuss the implications and meaning of "part of whole" for these instances. For example, ask, "Can you roll the sum ‘6' 13.2 times? Is that a good representation? Why or why not?"
Each group will have different quantities for each sum, because each experiment will vary slightly in outcomes. These differences can be explored later during the Explain portion of the lesson.
Explain
15 Minute(s)
After student pairs make their claims based on the scenarios from slide 17, ask open-ended questions to facilitate a class discussion. Allow students to reflect on their results before asking them to share aloud. This reflection time provides an opportunity for all to consider and formulate a response in order to contribute to the group discussion.
These are possible open-ended questions to help facilitate class discussion. Answers should come from the student pairs' experience with the previous activity.
What do you notice about your experimental probability results?
Which sums appeared most often during the experiment? Least often? Why do you think that occurred? Why do you think each group got different/similar answers?
What might happen to the results if we continued to roll the dice another 10 times, 15 times, 100 times, etc.? If we rolled it ____ many more times, how many times would you expect to get a sum that equals ____? (Students should use proportional reasoning to answer these questions, but, again, each group will have different answers based on the experimental data. Also, for the groups that don't receive a whole number, discuss the implication and meaning of part of a whole in these instances. For example, "Can you roll a sum 13.2 times?")
Think about the sample space of the sums created when using the two dice. What sums might you (theoretically) expect to see most/least often when considering the possible sums created between the two dice? How is theoretical probability different from experimental probability? (You might have to lead with questions like, What is the sample space of the sums? What is the theoretical probability of each sum? if students don't reach the connection on their own from the previous question.)
Considering the theoretical probability, how many times would you expect to roll a sum of ____ during 15 rolls?
Discuss the difference between sample space, theoretical probability, and experimental probability. (This is a great opportunity to refine students' thinking and connect back to academic vocabulary.)
Go to slide 18. Allow discussion for multiple students to share out about their experiences and findings.
Extend
Go to slide 19. Following the discussion, instruct students to think about other games or activities that they have at home that might have a sample space, theoretical probability, and experimental probability. As a whole group, brainstorm and list games or activities on the board. These values should be recorded in a shared digital document (Google Docs, Google Slides, etc.) linked in the attachments or provided on a handout for each student.
Go to slide 20. For homework, have students find sample space and theoretical probability using a game or activity that they have access to at home.
In class the next day, go to slide 21 and set up game stations around the classroom. (Optional: Ask students to bring the game or activity that they used for homework to school the next day so that their data for sample space and theoretically probability directly connect to the experimental data that they will record from the game stations). Students work in groups recording the experimental probability of the games that they choose to play.
In the same shared digital document used the night before, students should record their experimental probability findings for each game that they play. See the example below for what it might look like.
Evaluate
Go to slide 22. After all findings have been recorded, each student should individually reflect through a detailed writing using the strategy What Did I Learn Today. Writing should specifically focus on the connections (similarities and differences) between the experimental findings compared to the sample space and theoretical probability for each game listed or just the games they played in class. Students should also create and record examples of possible predictions based on the data from the experimental probability and the theoretical probability for each game (or just the games that they played in class).
Resources
Davis. B. (n.d.). Teaching mathematics through literature. LEARN North Carolina. http://web.archive.org/web/20180131132522/http://www.learnnc.org/lp/pages/3326?ref=search
Fandango Movieclips. [Movieclips]. (2012, Oct. 6). Jumanji (7/8) movie clip - earthquake (1995) HD [Video file]. YouTube. https://www.youtube.com/watch?v=SiUT8u1LckQ
Fandango Movieclips. [Movieclips]. (2012, Oct. 6). Jumanji (8/8) movie clip - Jumanji (1995) HD [Video file]. YouTube. https://www.youtube.com/watch?v=M0TjT53qpFY
K20 Center. (n.d.). Elbow partners. Strategies. https://learn.k20center.ou.edu/strategy/116
K20 Center. (n.d.). Think-pair-share. Strategies. https://learn.k20center.ou.edu/strategy/139
K20 Center. (n.d.). What did I learn today. Strategies. https://learn.k20center.ou.edu/strategy/169
Van Allsburg, C. (2011). Jumanji. Houghton Mifflin Harcourt.


